I wrote Chapter 9 in Enzymatic Fuel Cells: From Fundamentals to Applications, which is coming out May 19th. It’s edited by Plamen Atanassov, Heather Luckarift, and Glenn Johnson. The book grew out of a multi-university research project I was part of as a graduate student, with the goal of using biological catalysts for small power sources.

This is controversial research: it is, trust me. But if it works it has a high payoff. Summarizing just one possible application: we as humanity use an expensive element, platinum, for almost all of our room-temperature catalysis. This is why you don’t own very many things that involve room-temperature catalysis. However, living things do catalysis at physiological temperature (98.6 degrees F, not much higher) all the time. If we could use their tricks, catalysis would get much less expensive.

Some enzyme electrodes actually have incredibly high volumetric energy densities. The ones I was making as a graduate student reduced oxygen at 40 A/cm³ at 0.7 V, which is higher than some old-school platinum electrodes, and they do all the catalysis with copper. The downside is they don’t last a long time. But since platinum is 21,000 times more expensive than copper, it could be worth it.

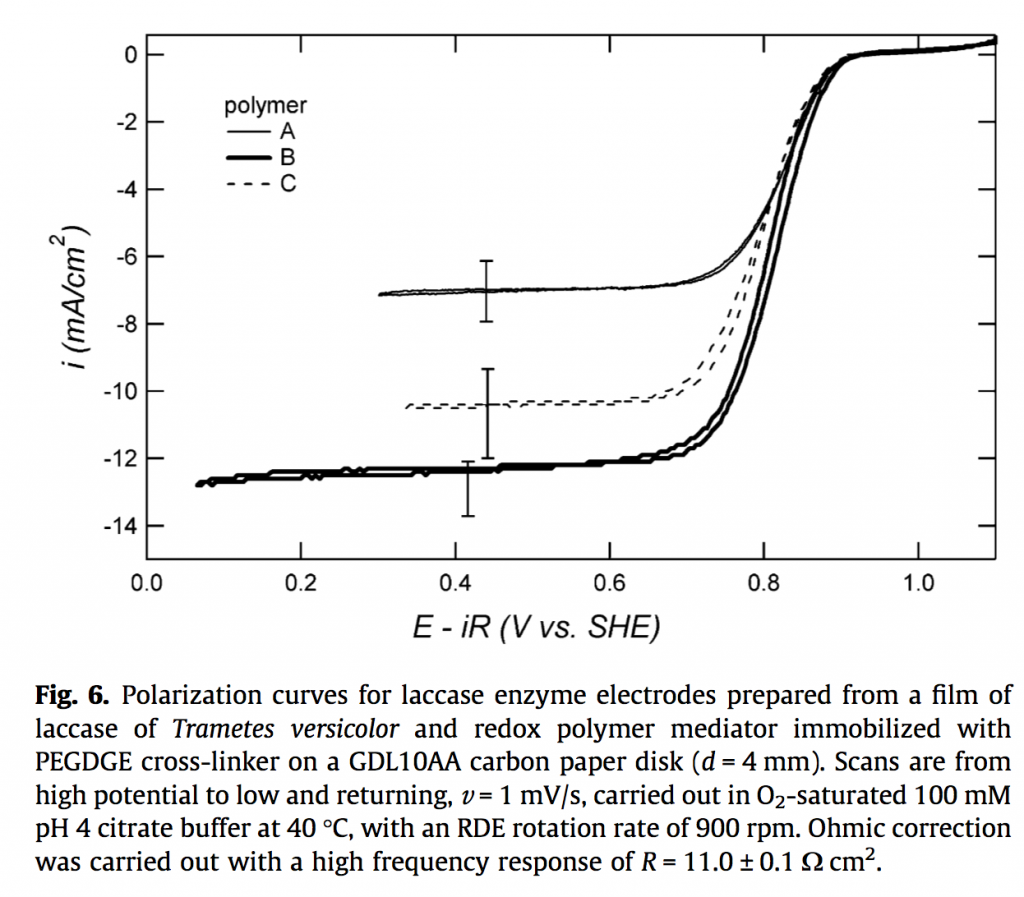
My chapter gave a me a chance to summarize my graduate research in a unified way, making the point I wanted to make. Essentially I showed you could double the catalytic current of one of these electrodes by being smarter about the transport phenomena involved. I published the results in two papers with dry, academic titles, because let’s face it I sometimes like the dry and academic. Only now, after a few more years of experience, I feel it would have been better to publish them together and title it The Point Is The Current Is Twice As High. Here’s the important graph below (from here):

See how the curves are S-shaped, and the bottom part is at about -7 mA/cm² in one case and about -14 mA/cm² in another? That’s the doubling. There’s actually a more important figure earlier in the paper, but this one is the easiest to explain. Science: it’s also about communication.